#C241118A. 树

标签（Label）

树的直径

网址（Website）

题目详情 - 树 - Super

题解 - 树 - Super

题目（Problem）

样例

输入数据 1

输出数据 1

1 1 2 4 6 6

【样例 1 解释】

$k = 4$ 时，联通块为 ${1}, {2}, {3}, {4,5,6}$。

输入数据 2

输出数据 2

1 1 3 5 5

【样例 2 解释】

$k = 3$ 时，联通块为 ${1,4,5}, {2}, {3}$。

【样例 3】

见选手目录下的 tree3.in 和 tree3.ans。

该样例满足子任务 $2$ 的限制。

【样例 4】

见选手目录下的 tree4.in 和 tree4.ans。

该样例满足子任务 $5$ 的限制。

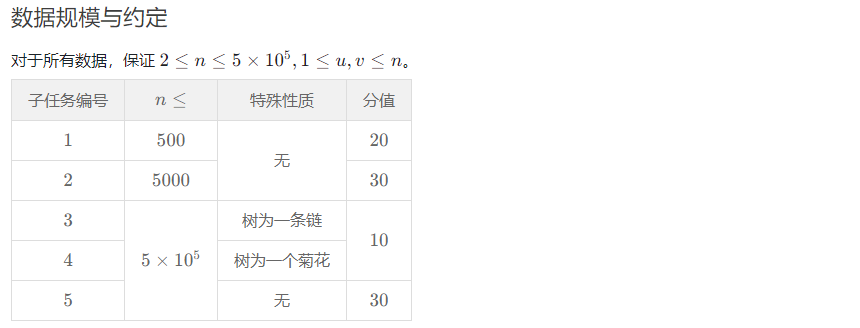
题解（Solution）

出题者题解

代码（Code）

#include<bits/stdc++.h>
#include<vector>
#include<queue>
#include<stack>
#define For(i,l,r) for(int i=l;i<=r;i++)
#define Rof(i,l,r) for(int i=l;i>=r;i--)
using namespace std;
#define P pair<int,int> 
#define int long long
#define x first
#define y second
inline int rd(){
	char c;bool f=false;while(!isdigit(c=getchar()))f=c=='-';int x=c^48;
	while(isdigit(c=getchar())){x=(((x<<2)+x)<<1)+(c^48);}return f?-x:x;
}
inline void write(int x){
	if(x<0) putchar('-'), write(-x);
	else if(x<10) putchar(x+'0');
	else write(x/10), putchar(x%10+'0');
}
const int inf = 0x3f3f3f3f3f3f3f3f;
const int N = 501234;
bool ST;
double Tim;

vector<int> ft[N];
int n,root1,root2,len;
int dis[N];

void dfs_dis(int x,int fa){
	dis[x] = dis[fa] + 1;
	for(auto y:ft[x]) if(y^fa) dfs_dis(y,x);
}

bitset<N> vst;
void dfs_vis(int x,int fa,int to,int step){
	if(x==to) return vst[x] = true, dis[x] = max(len-step, step), void();
	for(auto y:ft[x]) if(y^fa){
		dfs_vis(y,x,to,step+1);
		if(vst[y]) return vst[x] = true, dis[x] = max(step, len-step), void();
	}
}

void dfs_tag(int x,int fa){
	for(auto y:ft[x]) if(!vst[y]) vst[y]=true, dis[y] = dis[x]+1, dfs_tag(y,x);
}

int v[N],as[N];
int fa[N];inline int find(int x){
	if(x==fa[x]) return x;
	return fa[x] = find(fa[x]);
}

void Solve(){
	n=rd();For(i,1,n-1){
		int x=rd(),y=rd();
		ft[x].emplace_back(y);
		ft[y].emplace_back(x);
	}
	dis[0] = -1, dfs_dis(1,0);
	For(i,1,n) root1 = dis[root1]>dis[i] ? root1 : i;
	dis[0] = -1, dfs_dis(root1,0);
	For(i,1,n) root2 = dis[root2]>dis[i] ? root2 : i;
	len = dis[root2];//树的直径 
	dfs_vis(root1,0,root2,0);
	For(i,1,n) if(vst[i]) dfs_tag(i,0);
	
	For(i,1,n) v[dis[i]]++;
	int leijia = 0;
	Rof(i,n,1){
		leijia += v[i];
		as[i] = min(n-leijia+1, n);
	}For(i,1,n) write(as[i]), putchar(' ');
}

bool ED;
signed main(){
	cerr<<abs(&ST-&ED)/1024./1024.<<"MB\n";
	freopen("tree.in","r",stdin);
	freopen("tree.out","w",stdout);
	int Tt=1;Tim=clock();
	while(Tt--) Solve();
	return cerr<<"TIME:"<<(clock()-Tim)/CLOCKS_PER_SEC,0;
}